All students should graduate from high school ready for college, careers, and citizenship.

NOTE:
This sample of student work was selected from a special education classroom.
1. CCSS Alignment
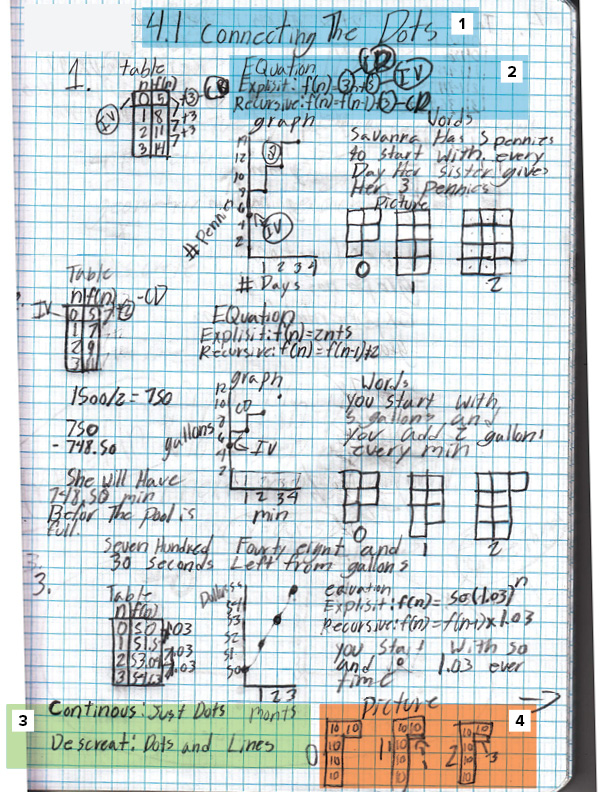
The student clearly can distinguish between situations that can be modeled by a linear function versus an exponential function. All tables show equal differences over equal intervals for linear situations and equal factors over equal intervals for exponential situations.
Standard referenced:
HSF-LE.A.1: Distinguish between situations that can be modeled with linear functions and with exponential functions.
2. CCSS Alignment
The student has clearly labeled the constant rate of change in the linear functions and the growth factor in the exponential functions. The student has identified the initial value and constant rate of change or constant growth factor in multiple representations. The student demonstrates an understanding of equal differences over equal intervals by their work for calculating how long it will take for the pool to fill in question 2. The student has also figured out that you can subtract 3% by multiplying by .97 in question 4.
Standard referenced:
HSF-LE.A.2: Construct linear and exponential functions, including arithmetic and geometric sequences, given a graph, a description of a relationship, or two input-output pairs (include reading these from a table).
3. Understanding
The student confuses the words discrete versus continuous, as shown by the explanation “continuous—just dots, discrete—dots and lines.”
4. Comprehension & Application
SMP.7: Look for and make use of structure.
The student is using multiple representations and is connecting the parameters for initial value and rate of growth in each situation across multiple representations. For example, the structure of the picture in question 3 clearly shows an understanding of exponential growth by the increasing area of the rectangle that gets added each minute.

1. Understanding
How is the student thinking about the exponential growth factor for both 1.03 in question 3 and 0.97 in question 4? How did the student arrive at these numbers? Was it from the table or an understanding of the relationship between subtracting 3% and how 0.97 models this?

1. CCSS Alignment
The student is working toward proficiency in HSF-LE.B.5 as shown in the student’s ability to interpret the parameters of a linear or exponential function given a context. The student was clearly able to create a linear function from the context given, but initially struggled to interpret the percent rate of change correctly as an exponential function.
Standard referenced:
HSF-LE.B.5: Interpret the parameters in a linear or exponential function in terms of a context.
2. Understanding
Because this is a special education student, when the work is compared to task 1, it appears that number size is obscuring the student’s ability to demonstrate proficiency in explicit and recursive equations. There is evidence of this by the revision marks on the student’s paper.
3. Comprehension & Application
SMP.7: Look for and make use of structure.
The student uses the table as a tool to reconsider the student’s initial interpretation of the context. The student was looking at the structure of the table with common factors and common differences, which allowed the student to look back and revise the interpretation.
4. CCSS Alignment
The intent of standard HSF-LE.A.3 is to recognize that exponential functions will eventually exceed a linear function. The student did make this observation. There is evidence that the table helped make this connection, even though there is no graphical evidence.
Standard referenced:
HSF-LE.A.3: Observe using graphs and tables that a quantity increasing exponentially eventually exceeds a quantity increasing linearly, quadratically, or (more generally) as a polynomial function.
